A Glossary for Geometric Morphometrics (part 2)
Continued from part 1.
L - The crucial matrix for computing the thin-plate spline
interpolant
between two landmark configurations. In this entry, k stands for the
number of landmarks, for historical reasons.
The equation of the thin-plate spline has coefficients
,
where h is a vector of the x- or y-coordinates
of the landmarks in a target form, followed by three 0’s (for two dimensional data,
four 0’s for three-dimensional data). The entries in the matrix L
are
wholly functions of the starting or reference form for the spline.
Bending energy is the upper k-by-k square of
.
For the complete formula for L, see the
Orange Book or
Rohlf (Black Book).
landmark - A specific point on a biological form or image
of a form located according to some rule. Landmarks with the
same name, homologues in the purely semantic sense, are presumed
to correspond in some sensible way over the forms of a data set.
See Type I, Type II, and
Type III landmarks.
least-squares estimates - Parameter estimates that minimize
the sum of squared differences between observed and predicted
sample values.
likelihood ratio test - A test based on the ratio of the
likelihood (the probability or density of the data given the parameters)
under a general model, to the likelihood when another, specified hypothesis
is true. Many of the commonly used statistical tests are likelihood
ratio tests, e.g., the t-test for comparisons of means,
Hotelling's , and the analysis of variance F-test.
linear combination - A sum of values each multiplied by
some coefficient. A linear combination can be expressed as the
inner product of two vectors, one representing the data and the
other a vector of coefficients.
linear transformation - In multivariate statistics, a linear
transformation is the construction of a new set of variables that
are all linear combinations of the original set. In geometric
morphometrics, one linear transformation takes Procrustes-fit
coordinates to partial warp scores; another takes them to relativewarp scores.
A linear transformation of a matrix A can
be written in the form y = Ax, where y is
the resulting linear combination of x, a column vector, with the rows of
A.
linear vector space - In morphometrics, the most common
k-dimensional linear vector space is the set of all real
k-dimensional vectors, including all sums of these vectors
and their scalar multiples. More generally, but informally, a
linear vector space is a set of elements, usually bits of geometry
or whole functions, that can be added together and can be multiplied
by real numbers in an intuitive way. The points of a plane don't
form a linear vector space (what is "five times a point"?),
but lines segments connecting all the points to the origin do
form such a space.
loading - The correlation or covariance of a measured variable with a linear
combination of variables. A loading is not the same as a coefficient.
In general, coefficients supply formulas for the computation
of scores whereas loadings are used for the biological interpretation
of the linear combination.
Mahalanobis distance - Also D or Mahalanobis D.
See generalized distance.
MANOVA - See multivariate analysis of variance.
maximum likelihood estimates - A likelihood function is
a probability or density function for a set of data and given
estimates of its parameters. A maximum likelihood estimate is
the set of parameter values that maximize this function. In
some cases, as with the arithmetic mean of a sample used as an
estimate of the parameter mu for a normally distributed population,
the maximum likelihood estimate may be identical to the least-squares
estimate.
median size - A size measure based on the repeated median
of interlandmark distances. Used in resistant-fit methods.
metric - A nonnegative function, dij,
of two points,
i and j, satisfying the following conditions:
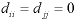 ,
if
,
if  then
then 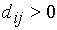 ,
,
 , and
, and 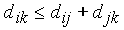 .
The last condition is known as the "triangle
inequality." The value of the function is referred to as
the "distance" between the two points. A space is called
"semimetric" if the distance between two points i and j can be equal to zero when
.
The last condition is known as the "triangle
inequality." The value of the function is referred to as
the "distance" between the two points. A space is called
"semimetric" if the distance between two points i and j can be equal to zero when
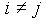 .
.
metric space - A space and a distance function defined on every pair of
points that meets the requirements of the definition of "metric" above.
morphometrics - From the Greek: "morph," meaning
"shape," and "metron," meaning "measurement."
Schools of morphometrics are characterized by what aspects of
biological "form" they are concerned with, what they
choose to measure, and what kinds of biostatistical questions
they ask of the measurements once they are made. The methods
of this glossary emphasize configurations of landmarks from whole
organs or organisms analyzed by appropriately invariant biometric
methods (covariances of taxon, size, cause or effect with position
in Kendall's shape space) in order to answer biological questions.
Another sort of morphometrics studies tissue sections, measures
the densities of points and curves, and uses these patterns to
answer questions about the random processes that may be controlling
the placement of cellular structures. A third, the method of
"allometry," measures sizes of separate organs and asks
questions about their correlations with each other and with measures
of total size. There are many others.
multiple discriminant analysis - Discriminant analysis
involving three or more a priori-defined groups.
See discriminant
analysis.
multiple regression - The prediction
of a dependent variable
by a linear combination of two or more independent variables using
least-squares methods for parameter estimation.
See multivariate regression and
multivariate multiple regression.
multivariate analysis of variance - MANOVA. An analysis
of variance of two or more dependent variables considered simultaneously.
multivariate morphometrics - A term
historically used for
the application of standard multivariate techniques to measurement
data for the purposes of morphometric analysis. Somewhat confusing
now as any morphometric technique must be multivariate in nature.
See traditional morphometrics.
multivariate multiple
regression - The prediction of two
or more dependent variables using two or more independent variables.
See multiple regression and
multivariate regression.
multivariate regression - The
prediction of two or more
dependent variables using one independent variable.
See multiple regression and
multivariate multiple regression.
normalize - To normalize a geometric object is to transform
it so that some function of its coordinates or other parameters has a prespecified
value. For example, vectors are often normalized by transformation
into unit vectors, which have length one.
nuisance parameters - Parameters of a model
that must be
fit but that are not of interest to the investigator. In morphometrics,
the parameters for translation and rotation are usually nuisance
parameters.
null model - The simplest model under consideration. The
null model for shape is the distribution in Kendall's shape space
that arises from landmarks that are distributed by independent
circular normal noise of the same variance in the original digitizing
plane or space and drawn from a single, homogeneous population.
It is exactly analogous to the usual assumption of "independent
identically distributed error terms" in conventional linear
models (regression, ANOVA).
oblique - At an angle that is not a multiple of 90 degrees.
Orange Book - Bookstein, F. L. 1991.
Morphometric Tools
for Landmark Data. Geometry and Biology. Cambridge University
Press: New York.
See also
Black Book,
Blue Book,
Red Book, and
Reyment's Black Book.
ordination - A representation of objects with respect to
one or more coordinate axes. There are many kinds of ordinations
depending upon the goals of the ordination and criteria used.
For example, plotting objects according to their scores on the
first two principal component axes provides the two-dimensional
ordination best summarizing the total variability of the objects
in the original sample space. Biplots combine an ordination of
specimens and an ordination of variables.
orthogonal - At right angles. In linear algebra, being
"at right angles" is defined relative to a symmetric matrix P,
such as the bending-energy matrix; two vectors x and y
are orthogonal with respect to P if xtPy=0.
Principal warps are orthogonal with respect to bending energy,
and relative warps are orthogonal with respect to
both bending energy
and the sample covariance matrix.
orthogonal superimposition - A superimposition using only
transformations that are all Euclidean similarities, i. e., involve
only translation, rotation, scaling, and, possibly, reflection.
orthonormal - A set of vectors is orthonormal if each has
length unity and all pairs are orthogonal with respect to some relevant matrix,
P, such as the identity matrix. A matrix is orthogonal
if its rows (columns) are orthonormal as a set of vectors.
outline - A mathematical curve that stands for the two-dimensional
image of a physical boundary. Outline data can be archived as
a sequence of point coordinates, but such points do not share
the notion of homology associated with landmarks (but see Sampson, 1996,
NATO volume "white book").
parameter - In general, a parameter is a number (an integer,
a decimal) indexing a function. For instance, the F-distribution
used to test decompositions of variance has two parameters, both
integers: the counts of the degrees of freedom for the two variances
whose ratio is being tested. In morphometrics, there are four
main kinds of parameters: nuisance parameters,
which must be
estimated to account for differences not of particular scientific
interest; the geometric parameters, such as shape coordinates,
in which landmark shape is expressed; statistical parameters,
such as mean differences or correlations, by which biological
interpretation is confronted with that data; and another set of
geometric parameters, such as partial warp scores
or
Procrustes residuals,
in which the findings of the statistical analysis are
expressed.
Partial Least Squares - Partial Least Squares is a multivariate
statistical method for assessing relationships among two or more
sets of variables measured on the same entities. Partial Least
Squares analyses the covariances between the sets of variables
rather than optimizing linear combinations of variables in the
various sets. Their computations usually do not involve the inversion
of matrices (see the Orange Book).
partial warp scores - Partial warp scores
are the quantities
that characterize the location of each specimen in the space of
the partial warps. They are a rotation of the Procrustes
residuals
around the Procrustes mean configuration. For the nonuniform partial warps,
the coefficients for
the rotation are the principal warps,
applied first to the x-coordinates
of the Procrustes residuals, then to the
y-coordinates
and, for three-dimensional data, the z-coordinates.
Coefficients for the uniform partial warps are produced by special formulas
(see Bookstein's "Uniform" chapter, NATO volume "white book").
partial warps - Partial warps are an auxiliary structure
for the interpretation of shape changes and shape variation in
sets of landmarks. Geometrically, partial warps are an orthonormal
basis for a space tangent to Kendall's shape space. Algebraically,
the partial warps are eigenvectors of the
bending energy matrix
that describes the net local information in a deformation along
each coordinate axis. Except for the very largest-scale partial
warp, the one for uniform shape change, they have an approximate
location and an approximate scale.
precision - The closeness of repeated measurements
to the
same value. See accuracy.
preform space - The space corresponding to centered objects,
i. e., differences in location have been removed. It is of k(p-1)
dimensions.
preshape space - The space corresponding to figures that
have been centered and scaled but not rotated to alignment. It
is of k(p-1)-1 dimensions.
principal axes and strains - A change of one triangle into
another, or of one tetrahedron into another, can be modelled as
an affine transformation which can be parameterized by its effect
on a circle or sphere.
An affine transformation takes circles
into ellipses. The principal axes of the shape change are the
directions of the diameters of the circle that are mapped into
the major and minor axes of the ellipse. The principal strains
of the change are the ratios of the lengths of the axes of the
ellipse to the diameter of the circle. In the case of the tetrahedron,
there are three principal axes, the axes of the ellipsoid into
which a sphere is deformed. One has the greatest principal strain
(ratio of axis length to diameter of sphere), one the least, and
there is a third perpendicular to both, having an intermediate
principal strain.
principal components analysis - The eigenanalysis of the
sample covariance matrix. Principal components (PC's) can be
defined as the set of vectors that are orthogonal both with respect
to the identity matrix and the sample covariance matrix.
They can also be defined sequentially: the first is the linear
combination with the largest variance of all those with coefficients
summing in square to 1; the second has the largest variance
(when normalized that way) of all that are uncorrelated with the
first one; etc. One way to compute principal components is to
use a singular value decomposition. Relative warps are principal
components of partial warp scores. There is a lot to be said
about PC's; see any of the colored books.
principal warps - Principal warps are
eigenfunctions of
the bending-energy matrix interpreted as actual warped surfaces
(thin-plate splines) over the picture of the original landmark
configuration. Principal warps are like the harmonics in a
Fourier
analysis (for circular shape) or Legendre polynomials (for linear
shape) in that together they decompose the relation of any sample
shape to the sample average shape as a unique summation of multiples
of eigenfunctions of bending energy. They differ from these
more familiar analogues in that there are only p-3 of them for
a set of p 2D landmarks (p-4 for 3D data) - they form a finite series. Together with
the uniform terms, the partial warps, which are projections (shadows)
of the principal warps, supply an orthonormal basis for a space
that is tangent to Kendall's shape space in the vicinity of a
mean form.
Procrustes distance -
Approximately (see Bookstein's "Combining"
chapter, NATO volume "white book"), the square root of the sum of squared differences
between the positions of the landmarks in two optimally (by least-squares)
superimposed configurations at centroid size. This is the distance that defines
the metric for
Kendall's shape space.
Procrustes mean - The shape that has the least summed squared
Procrustes distance to all the configurations of a sample; the
best choice of consensus configuration for most subsequent morphometric
analyses (see Bookstein's "Combining" chapter, NATO
volume "white book").
Procrustes methods - A term for least-squares methods for
estimating nuisance parameters of the Euclidean similarity transformations.
The adjective "Procrustes"
refers to the Greek giant who would stretch or shorten victims
to fit a bed and was first used in the context of superimposition
methods by Hurley and Cattell, 1962, The Procrustes program: producing
a direct rotation to test an hypothesized factor structure, Behav.
Sci. 7:258-262.
Modern workers have often cited Mosier (1939), a psychometrician, as the earliest known
developer of these methods. However, Cole (1996) reports that Franz Boas in 1905
suggested the "method of least differences" (ordinary Procrustes analysis) as a means
of comparing homologous points to address obvious problems with the
standard point-line registrations (Boas, 1905). Cole further points out
that one of Boas' students extended the method to the construction of mean
configurations from the superimposition of multiple specimens using
either the standard registrations of Boas' method (Phelps, 1932).
The latter being essentially a Generalized Procrustes Analysis.
References:
Cole, T. M. 1996. Historical note: early anthropological contributions to "geometric morphometrics."
Amer. J. Phys. Anthropol. 101:291-296.
Boas, F. 1905. The horizontal plane of the skull and the general problem of the
comparision of variable forms. Science, 21:862-863.
Phelps, E. M. 1932. A critique of the principle of the horizontal plane of the skull.
Amer. J. Phys. Anthropol., 17:71-98.
Mosier, 1939, Determining a simple structure when loadings
for certain tests are known, Psychometrika 4:149-162.
Procrustes residuals - The set of
vectors connecting the
landmarks of a specimen to corresponding landmarks in the consensus
configuration after a Procrustes fit. The sum of squared lengths
of these vectors is approximately the squared Procrustes distance
between the specimen and the consensus in
Kendall's shape space.
The partial warp scores are an orthogonal rotation of the full set of these
residuals.
Procrustes scatter - A collection of forms all superimposed
by ordinary orthogonal Procrustes fit over one single consensus
configuration that is their Procrustes mean; a scatter of all
the Procrustes residuals each centered at the corresponding landmark
of the Procrustes mean shape.
Procrustes superimposition - The construction of a two-form
superimposition by least squares using orthogonal or affine transformations.
Red Book - Bookstein, F. L., B. Chernoff, R. Elder, J.
Humphries, G. Smith, and R. Strauss. 1985. Morphometrics in
Evolutionary Biology. Special Publication No. 15, Academy
of Natural Sciences: Philadelphia.
See also Black Book,
Blue Book,
Orange Book, and
Reyment's Black Book.
reference configuration - In the context of superimposition methods,
this is the configuration to which
data are fit. It may be another specimen in the sample but usually it will be
the average (consensus) configuration for a sample.
The construction of two-point shape coordinates
does not involve a reference
specimen, though the intelligent choice of baseline for the construction usually does.
The reference configuration corresponds to the point of tangency of the
linear tangent space used to approximate Kendall's shape space.
The mean configuration is usually used
as the reference in order to minimize distortions caused by this approximation.
When splines and warps are part of the analysis,
the bending energy that goes with them is computed using the
geometry of the grand mean shape, and the orthogonality that
characterizes the partial warps is with respect to this particular
formula for bending energy.
There has been some controversery regarding the choice of reference. See the following
papers.
Rohlf, F. James. 1998. On applications of geometric morphometrics
to studies of ontogeny and phylogeny. Systematic Biology, 47:147-158.
Adams, D. C. and M. S. Rosenberg. 1998. Partial warps, phylogeny, and ontogeny:
a comment on Fink and Zelditch (1995). Systematic Biology, 47:168-173.
Zelditch, M. L., W. L. Fink, D. L. Swiderski, and B. L. Lundrigan. 1998.
On applications of geometric morphometrics to studies of ontogeny and phylogeny:
a reply to Rohlf. Systematic Biology, 47:159-167.
Zelditch, M. L. and W. L. Fink. 1998. Partial warps, phylogeny and ontogeny:
a reply to Adams and Rosenberg. Systematic Biology, 47:345-348.
regression - A model for predicting one variable from another.
Due to Francis Galton, the word comes from the fact that when
measurements of offspring, whether peas or people, were plotted
against the same measurements of their parents, the offspring
measurements "went back" or regressed towards the mean.
relative warps - Relative warps are principal components
of a distribution of shapes in a space tangent to Kendall's shape
space. They are the axes of the "ellipsoid" occupied
by the sample of shapes in a geometry in which spheres are defined
by Procrustes distance. Each relative warp, as a direction of
shape change about the mean form, can be interpreted as specifying
multiples of one single transformation, a transformation that
can often be usefully drawn out as a thin-plate spline. In a
relative warps analysis, the parameter
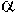 can be used to weight shape variation by the geometric scale of shape
differences. Relative warps can be computed from Procrustes residuals
or from partial warps (see Bookstein's "Combining" chapter,
NATO volume "white book").
can be used to weight shape variation by the geometric scale of shape
differences. Relative warps can be computed from Procrustes residuals
or from partial warps (see Bookstein's "Combining" chapter,
NATO volume "white book").
repeated median - A median of medians. Repeated medians
are used to estimate some superimposition parameters in the resistant-fit
methods. For example, the resistant-fit rotation estimate is the
median of the estimates obtained for each landmark, which is,
in turn, the median of angular differences between the reference
configuration and the configuration being fit of the line segments
defined using that landmark and the other n-1 landmarks.
Repeated medians are insensitive to larger subsets of extremely
deviant values than simple medians.
residual - The deviations of an observed value or vector of values from some
expectation, e.g., the differences between a shape and its prediction
by an allometric regression expressed in any set of shape coordinates.
resistant-fit superimposition - Superimposition methods
that use median- and repeated-median-based estimates of fitting
parameters rather than least-squares estimates. Resistant-fit
procedures are less sensitive to subsets of extreme values than
those of comparable least-squares methods. As such, their results may
provide a simple description of differences in shape that are
due to changes in the positions of just a few landmarks. However,
resistant-fit methods lack the well-developed distributional theory
associated with the least-squares fitting methods. See Slice, 1996,
NATO volume "white book".
resolution - The smallest scale distinguishable by a digitizing,
imaging, or display device.
Reyment's Black Book -
Reyment, R. A. 1991. Multidimensional
Palaeobiology. Pergamon Press: Oxford.
See also Black Book,
Blue Book,
Orange Book, and Red Book.
ridge curve - Ridge curves are curves on a surface along
which the curvature perpendicular to the curve is a local
maximum. For instance on a skull, the line of the jaw or the rim
of an orbit. See Dean, 1996, NATO volume "white book".
rigid rotation - An orthogonal transformation of a real vector space
with respect to the Euclidean distance metric.
Such transformations
leave distances between points and angles between vectors unchanged.
A principal components analysis represents a rigid rotation to
new orthogonal axes. A canonical variates analysis
does not.
score - A linear combination of an observed set of measured
variables. The coefficients for the linear combination are usually
determined by some matrix computation. Multivariate statistical
findings in the form of coefficient vectors can usually be more
easily interpreted if scores are also shown case by case, their
scatters, their loadings (correlations with the original variables),
etc.
shape - The geometric properties of a configuration of
points that are invariant to changes in translation, rotation,
and scale. In morphometrics, we represent the shape of an object
by a point in a space of shape variables, which are measurements
of a geometric object that are unchanged under similarity transformations.
For data that are configurations of landmarks, there is also
a representation of shapes per se, without any
nuisance parameters
(position, rotation, scale), as single points in a space,
Kendall's
shape space, with a geometry given by
Procrustes distance. Other
sorts of shapes (e.g., those of outlines, surfaces, or functions)
correspond to quite different statistical spaces.
shape coordinates - In the past, any system of distance-ratios
and perpendicular projections permitting the exact reconstruction
of a system of landmarks by a rigid trusswork. Now, more generally,
coordinates with respect to any basis for the tangent space to
Kendall's shape space in the vicinity of a mean form: see
Procrustes residuals,
partial warp scores,
two-point shape coordinates.
shape space - A space in which the shape of a figure
is represented by a single point. It is of 2p-4
dimensions for 2-dimensional coordinate data and 3p-7 dimensions
for 3-dimensional coordinate data.
See Kendall's shape space.
shape variable - Any measure of the geometry of a biological
form, or the image of a form, that does not change under similarity
transformations: translations, rotations, and changes of geometric
scale (enlargements or reductions).
Useful shape variables include angles, ratios of distances, and
any of the sets of shape coordinates that arise in geometric morphometrics.
shear -
In two-dimensional problems, shape aspects of
any affine transformation can be diagrammed as a pure shear, a map
taking a square to a parallelogram of unchanged base segment and
height.
This is a transformation that
leaves one Cartesian coordinate, y, invariant and alters the other by a
translation that is a multiple of y: for instance, what happens
when you slide the top of a square sideways without altering its
vertical position or the length of the horizontal edges. The score
for such a translation,
together with a separate score for change
in the horizontal/vertical ratio,
supplies one orthonormal basis for the
subspace of uniform shape changes of two-dimensional data.
Without the adjective "pure," geometric morphometricians usually use
the word "shear" as an informal synonym for "affine transformation,"
since any 2D uniform transformation can be drawn as one
if you wish.
In multivariate morphometrics, a somewhat different use of pure
shear is in a transformation of the "shape principal components"
of an allometric analysis of distances to be uncorrelated with
within-group size (see refs).
See Bookstein et al. (1985)
for a description of the method of shearing and the critique by Rohlf & Bookstein (1987)
of the technique as a method of size correction.
References:
Bookstein, F. L., B. Chernoff, R. Elder, J.
Humphries, G. Smith, and R. Strauss. 1985. Morphometrics in
Evolutionary Biology. Special Publication No. 15, Academy
of Natural Sciences: Philadelphia.
Rohlf, F. James and Bookstein, F. L. 1987. A comment on shearing as a method for "size correction".
Systematic Zoology, 36:356-367.
similarity transformation - A change of Cartesian coordinate
system that leaves all ratios of distances unchanged.
The term proper or special similarity group of similarities is sometimes used
when the transformations do not involve reflection.
Similarities
are arbitrary combinations of translations, rotations, and changes
of scale. Compare affine transformation.
singular value decomposition -
Any mxn matrix X may
be decomposed into three matrices U, D, V
(with dimensions mxm,
mxn,
and nxn, respectively)
in the form: X=UDVt, where the columns of U
are orthogonal, D is a diagonal matrix of singular values,
and the columns of V are orthogonal. The singular value
decomposition of a variance-covariance matrix S is written
as S=ELEt, where L is the diagonal matrix
of eigenvalues and E the matrix of eigenvectors.
size measure - In general, some measure of a form (i. e.,
an invariant under the group of isometries) that scales as a positive
power of the geometric scale of the form. Interlandmark lengths
are size measures of dimension one, areas are size measures of
dimension two, etc.
space - In statistics, a collection of objects or measurements
of objects, treated as if they were points in a plane, a volume,
on the surface of a sphere, or on any higher-dimensional generalization
of these intuitive structures. Examples are: Euclidean spaces,
sample spaces, shape spaces, linear vector spaces, etc.
superimposition - The transformation of one or more figures
to achieve some geometric relationship to another figure. The
transformations are usually affine transformations or similarities.
They can be computed by matching two or three landmarks, by least-squares
optimization of squared residuals at all landmarks, or in other
ways. Sometimes informally referred to as a "fit" or
"fitting," e.g., a resistant fit.
SVD - See singular value decomposition.
T2 statistic - A multivariate generalization
of the univariate t2 statistic. It is the square of the
ratio of the group mean difference to the standard error of that
difference. Used in the T2-test.
T2-test - A test due to Hotelling for comparing
an observed mean vector to a parametric mean; or comparing the
difference between two mean vectors to a parametric difference
(usually the zero vector). If the observations are independently multivariate
normal, then the T2-test may be used to test null hypotheses
using the F-distribution. T2 is also closely related
to Mahalanobis D2. See Marcus (Black Book).
tangent space - Informally, if S is a curving space and P a point in it,
the tangent space to S at P is a linear space T
having points with the same "names" as the points in S and
in which the metric on
S "in the vicinity of P" is very nearly the ordinary Euclidean
metric on T.
One can visualize T as the projection of S onto a
"tangent plane" "touching" at P
just like a map is a projection of the surface of the earth onto flat paper.
In geometric morphometrics, the most relevant tangent space is
a linear vector space that is tangent to Kendall's shape space
at a point corresponding to the shape of a reference configuration
(usually taken as the mean of a sample of shapes). If variation
in shape is small then Euclidean distances in the tangent space
can be used to approximate Procrustes distances in Kendall's shape
space. Since the tangent space is linear, it is possible to apply
conventional statistical methods to study variation in shape.
See Rohlf, 1996, NATO volume "white book", and Bookstein’s 1996 "Combining" chapter (NATO volume "white book").
tensor - An example of a tensor in morphometrics is the
representation of a uniform component of shape change as a transformation
matrix. The transformation matrix assigns to each vector in a
starting (or average) form a vector in a second form. A rigorous,
general definition of a tensor would be beyond the scope of this
glossary, but a reasonably intuitive characterization comes from
Misner, Thorne, and Wheeler, Gravitation (Freeman, 1973):
a tensor is a "geometric machine" that is fed one or
more vectors in an arbitrary Cartesian coordinate system and that
produces scalar values (ordinary decimal numbers) that are independent
of that coordinate system. In morphometrics, these "numbers"
will be ordinary geometric entities like lengths, areas, or angles:
anything that doesn't change when the coordinate system changes.
For the representation of a uniform component as a transformation
matrix, the "scalars" of the Misner-Thorne-Wheeler metaphor
are the lengths of the resulting vectors and the angles among
them.
A different tensor representing the same uniform transformation
is the relative metric tensor, which you probably know
as the ellipse of principal axes and principal strains. This
tensor produces the necessary numerical invariants (distances
in the second form as a function of coordinates on the first form)
directly. Other tensors include the metric tensor of a
curving surface which expresses distance on the surface as a function
of the parameters in which surface points are expressed and the
curvature tensor of the same surface which expresses the
way in which the surface "falls away" from its tangent
plane at any point.
thin-plate spline - In continuum mechanics, a thin-plate
spline models the form taken by a metal plate that is constrained
at some combination of points and lines and otherwise free to
adopt the form that minimizes bending energy. (The extent of
bending is taken as so small that elastic energy - stretches and
shrinks in the plane of the original plate - can be neglected.)
One particular version of this problem - an infinite, uniform
plate constrained only by displacements at a set of discrete points
- can be solved algebraically by a simple matrix inversion. In
that form, the technique is a convenient general approach to the
problem of surface interpolation for computer graphics and computer-aided
design. In morphometrics, the same interpolation (applied once
for each Cartesian coordinate) provides a unique solution to the
construction of D'Arcy Thompson-type deformation grids for data
in the form of two landmark configurations.
traditional morphometrics -
Application of multivariate
statistical methods to arbitrary collections of size or shape variables such
as distances and angles. "Traditional morphometrics"
differs from the geometric morphometrics discussed here in that
even though the distances or measurements are defined to record biologically
meaningful aspects of the organism, but the geometrical relationships
between these measurements are not taken into account. Traditional
morphometrics makes no reference to Procrustes distance or any
other aspect of Kendall's shape space.
See multivariate morphometrics and geometric morphometrics
transformation - In general, a replacement of landmark
coordinates by another set purporting to pertain to the same landmarks.
For example, a matrix of landmark coordinates might be transformed
by multiplication by another matrix to produce a new set of coordinates
that have been scaled, rotated, and translated with respect to
the original data.
two-point shape coordinates - A convenient system of shape
coordinates, originally Francis Galton's, rediscovered by Bookstein,
consisting (for two-dimensional data) of the coordinates of landmarks
3, 4, ... after forms are rescaled and repositioned so that landmark
1 is fixed at (0,0) and landmark 2 is fixed at (1,0) in a Cartesian
coordinate system. Also referred to as Bookstein coordinates
or Bookstein's shape coordinates.
Type I landmark - A
mathematical point whose claimed homology
from case to case is supported by the strongest evidence, such
as a local pattern of juxtaposition of tissue types or a small
patch of some unusual histology.
Type II landmark - A
mathematical point whose claimed homology
from case to case is supported only by geometric, not histological,
evidence: for instance, the sharpest curvature of a tooth.
Type III landmark - A
landmark having at least one deficient
coordinate, for instance, either end of a longest diameter, or
the bottom of a concavity. Type III landmarks characterize more
than one region of the form. The multivariate machinery of geometric
morphometrics permits them to be treated as landmark points in
some analyses, but the deficiency they embody must be kept in
mind in the course of any geometric or biological interpretation.
unbiased estimator - An
estimator,
 ,
that has as its expected
value the parametric value, q, it is intended to estimate:
,
that has as its expected
value the parametric value, q, it is intended to estimate:
 .
See consistent estimator
and asymptotically
unbiased estimator.
.
See consistent estimator
and asymptotically
unbiased estimator.
uniform shape component - That part of the difference in
shape between a set of configurations that can be modeled by
an affine transformation.
Once a metric is supplied for shape
space one can ascertain which such transformation takes a reference
form closest to a particular target form. For the Procrustes
metric (the geometry of Kendall's shape space), that uniform transformation
is computed by a formula based in
Procrustes residuals
or by another
based in two-point shape coordinates
(see Bookstein's 1996 "Uniform"
chapter, NATO volume "white book"). Together with the partial warps, the uniform
component defined in this way supplies an orthonormal basis for
all of shape space in the vicinity of a mean form. In this setting,
the uniform shape component may also be interpreted as the projection
of a shape difference (between two group means, or between a mean
and a particular specimen) into the plane (or hyperplane for data
of dimension greater than two) through that mean form and all
nearby forms related to it by affine transformations. For descriptive
purposes, the uniform component is parameterized not by a vector,
like the partial warps, but by a representation as a tensor, in
terms of sets of shears and dilations with respect to a fixed,
orthogonal set of Cartesian axes.
weight matrix , W matrix -The matrix of partial warp scores,
together with the uniform component, for a sample of shapes.
The weight matrix is computed as a rotation of the Procrustes-residual
shape coordinates; like them, they are a set of shape coordinates
for which the sum of squared differences is the squared Procrustes
distance between any two specimens.
Wright factor analysis - A version of factor analysis, due to Sewall Wright,
in which a path model is used to describe the relation
between the measured variables and the factors of interest. It
is usually exploratory, in that one fits a simple one factor model
iteratively to maximally explain the correlations among variables,
and then proceeds to find additional factors to fit to the residuals,
and so on until the data is adequately fit.
See the Orange book
for examples and discussion of the application of this approach
to the analysis of size and group factors for morphometric data.
z - Notation for complex numbers in two-dimensional Procrustes
formulas.
Acknowledgments
Work on this glossary by Slice and Rohlf was been supported by
grants BSR-89-18630 and DEB-93-17572 from the Systematic Biology
Program of the National Science Foundation.
Bookstein's work in morphometrics is supported by NIH grants DA-09009
and GM-37251. The former of these is jointly supported by the
National Institute on Drug Abuse, the National Institute of Mental
Health, and the National Institute on Aging as part of the Human
Brain Project.
This is publication number 944 from the Graduate Studies in Ecology
and Evolution, State University of New York at Stony Brook.
References
Bookstein, F. L. 1991. Morphometric tools for landmark data. Geometry and biology.
Cambridge University Press: New York.
Bookstein, F. L., B. Chernoff, R. Elder, J. Humphries, G. Smith, and
R. Strauss. 1985.
Morphometrics in evolutionary biology: The geometry of size and shape change,
with examples from fishes. Academy of Natural Sciences of Philadelphia
Special Publication 15.
Bookstein, F. L., and W. D. K. Green. 1993. A feature space for edgels in images
with landmarks. Journal of Mathematical Imaging and Vision 3:231–261.
Hurley and Cattell. 1962. The Procrustes program: Producing a direct rotation to test
an hypothesized factor structure. Behavior Science 7:258–262.
Lele, S., and J. T. Richtsmeier. 1991. Euclidean distance matrix analysis:
A coordinate free approach for comparing biological shapes using landmark data,
American Journal of Physical Anthropology 86:415-428.
Marcus, L. F., E. Bello, and A. García-Valdecasas (eds.). 1993.
Contributions to morphometrics. Monografias del Museo Nacional de
Ciencias Naturales 8, Madrid.
Misner, C., K. Thorne, and J. Wheeler. 1973. Gravitation. Freeman: New York.
Mosier, C. I. 1939. Determining a simple structure when loadings for
certain tests are known.
Psychometrika 4:149–162.
Reyment, R. A. 1991. Multidimensional palaeobiology. Pergamon Press: Oxford.
Reyment, R. A., and K. G. Jöreskog. 1993. Applied factor analysis in the
natural sciences.
Cambridge University Press: Cambridge.
Rohlf, F. J., and F. L. Bookstein (eds.). 1990. Proceedings of the
Michigan morphometrics workshop.
University of Michigan Museum of Zoology Special Publication 2.
Revised November 24, 1998 by F. James Rohlf
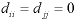 ,
if
,
if  then
then 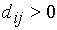 ,
,
 , and
, and 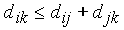 .
The last condition is known as the "triangle
inequality." The value of the function is referred to as
the "distance" between the two points. A space is called
"semimetric" if the distance between two points i and j can be equal to zero when
.
The last condition is known as the "triangle
inequality." The value of the function is referred to as
the "distance" between the two points. A space is called
"semimetric" if the distance between two points i and j can be equal to zero when
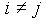 .
.
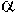
 ,
that has as its expected
value the parametric value, q, it is intended to estimate:
,
that has as its expected
value the parametric value, q, it is intended to estimate:
 .
See
.
See